Mahi Tuatahi

\[ \begin{aligned} F=ma \end{aligned} \]

1. State what each letter stands for
2. Give the units for each letter
3. Rearrange the equation for \(m\) and \(a\)
4. Using the formula, what are the SI units for F (not Newtons)?

For a car of mass 1500kg which is accelerating at \(3.7ms^{-2}\):

1. What net force is needed to maintain this acceleration?

\[ \begin{aligned} & && \text{Knowns} \cr & && \text{Unknowns} \cr & && \text{Formula} \cr & && \text{Sub and Solve} \end{aligned} \]

2. If the engine is producing \(6000N\) of thrust, why is this different from your calculation?

Torque (\(\tau\)) – Tōpana Whakahuri

Torque can be thought of as the turning effect around a pivot. Torque is sometimes known as moment or leverage.

\[ \begin{aligned} \tau &= Fd_{\bot} \cr torque &= Newtons \times metres \cr torque &= \text{Newton meters (Nm)} \end{aligned} \]

• \(F =\) force in Newtons
• \(d_{\bot} =\) perpendicular distance of force from pivot

Torque (\(\tau\)) – Tōpana Whakahuri

• A small force at a small distance produces a small torque,
• the same small force at a larger distance produces a larger torque.
• The force is in a straight line
• The torque is circular (CW or CCW)

Pātai: Individual Torques

\[ \begin{aligned} & && \text{Knowns} \cr & && \text{Unknowns} \cr & && \text{Formula} \cr & && \text{Sub and Solve} \end{aligned} \]

Pātai Whā: Does torque have a direction?

Yes, and you must always state which direction it is acting in.

Clockwise or Anticlockwise

Torque & Equilibrium

Equilibrium, Expanded

Newton’s First Law tells us equilibrium is when an object is at rest or moving uniformly (\(F_{net}=0\)).

For this to occur we need two things to be true. You must state these assumptions when doing any equilibrium question.

1. Sum of all forces to be 0 (\(\sum F = 0N\))
2. Sum of all torques to be 0 (\(\sum\tau = 0Nm\))

Pātai Tahi

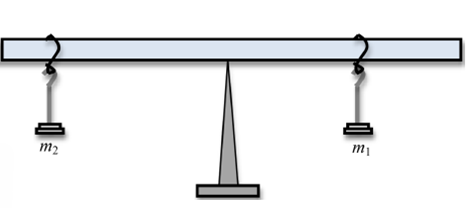
• \(m_{1}=2kg\), \(d_{1}=15cm\), \(m_{2}=1kg\), \(d_{2}=30cm\)
• Calculate the clockwise and anticlockwise torques
• Is the system in equilibrium?

Pātai Rua

• \(m_{1}=7kg\), \(d_{1}=36cm\), \(m_{2}=13kg\), \(d_{2}=65cm\)
• Calculate the clockwise and anticlockwise torques
• Is the system in equilibrium?

Torque & Equilibrium

The plank may not be massless. You may need to take it into account.

• The mass of the plank acts through its centre of gravity
• Because the plank is uniform, this is the middle of the plank

How To Solve A Torque Problem

1. Draw and label all forces on a diagram
2. Draw and label the distances between all forces and the pivot
3. Calculate all clockwise torque
4. Calculate all anticlockwise torque
5. Balance torques & forces
6. Find the unknown value

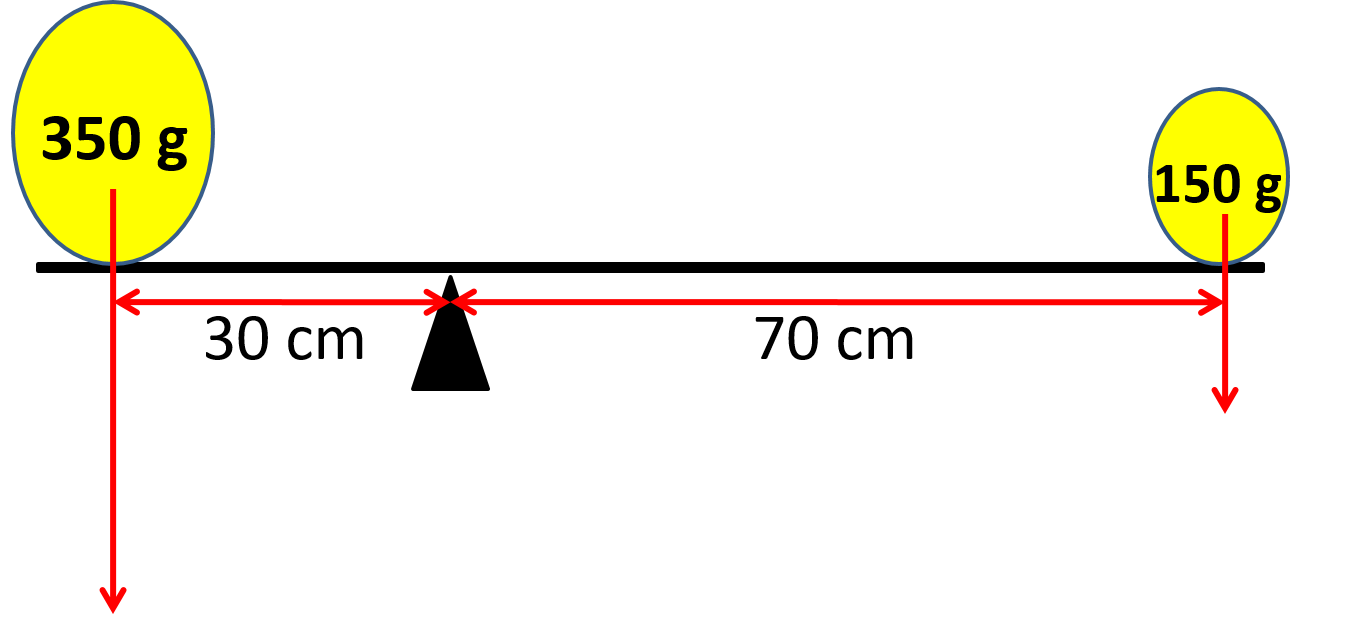
Pātai: Balanced or Unbalanced?

• \(d_{1}=30cm\), \(d_{2}=70cm\), \(m_{1}=900g\), \(m_{2}=300g\), see-saw mass = \(100g\).
• Is the system in equilibrium?
• Step 1: Calculate the total anticlockwise moment
• Step 2: Calculate the total clockwise moment
• Check if they are balanced.

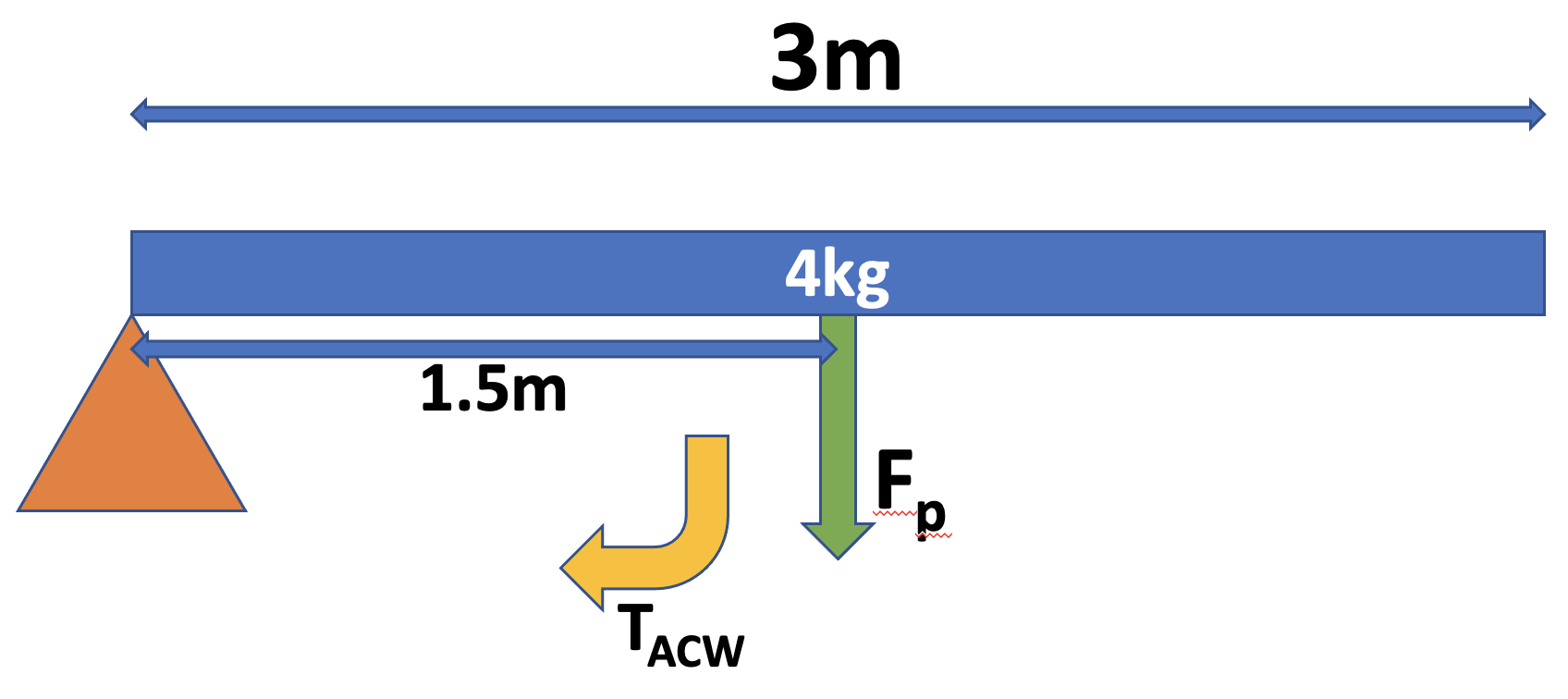
Pātai: Unknown Force

• Assume the system is in equilibrium (\(\tau_{clockwise} = \tau_{anticlockwise}\))
• \(d_{1}=0.5m\), \(d_{2}=1.5m\), \(F_{1}=2.5N\), see-saw mass = \(0.5kg\), \(F_{2}=?\).
• Draw the weight force of the see-saw on your diagram
• Find the unknown force, \(F_{2}\)

Whakatika

\[ \begin{aligned} \tau_{CW} &= \tau_{ACW} \cr \tau_{p} + \tau_{2} &= \tau_{1} \cr F_{p}d_{p} + F_{2}d_{2} &= F_{1}d_{1} \cr ((0.5 \times 9.8)\times 0.5) + F_{2}\times 1.5 &= 2.5 \times 0.5 \cr 2.45 + F_{2} \times 1.5 &= 1.25 \cr F_{2} &= \frac{1.25 - 2.45}{1.5} = -0.8N \end{aligned} \]

This is an interesting answer - it implies that \(F_{2}\) is actually acting up.

Pātai: Finding the Supports

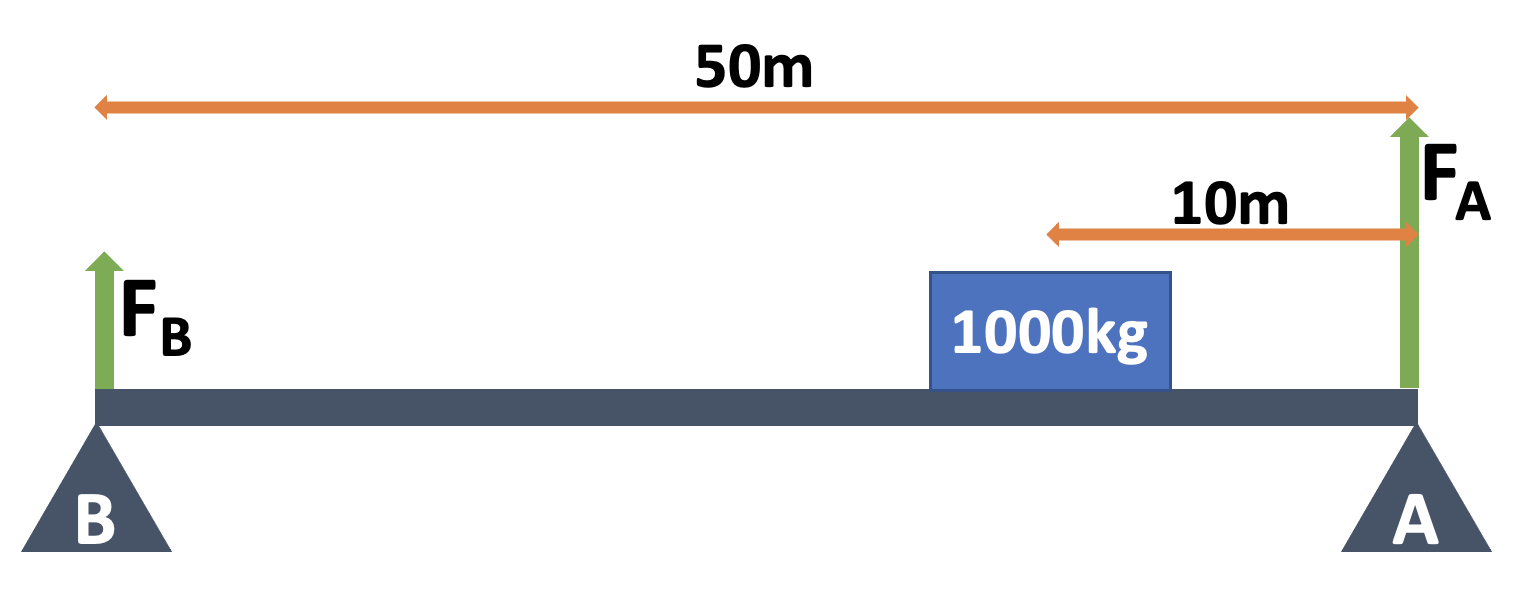
• Given that the bridge weighs \(10,000kg\), find the support force provided by A and B.
  • To find A, we make B the pivot. We also ignore \(F_{B}\) because it acting at the pivot and cannot provide any rotational forces.
  • To find B, we make A the pivot. We also ignore \(F_{A}\) because it acting at the pivot and cannot provide any rotational forces.
• Solve for each support independently.

Whakatika: Support A

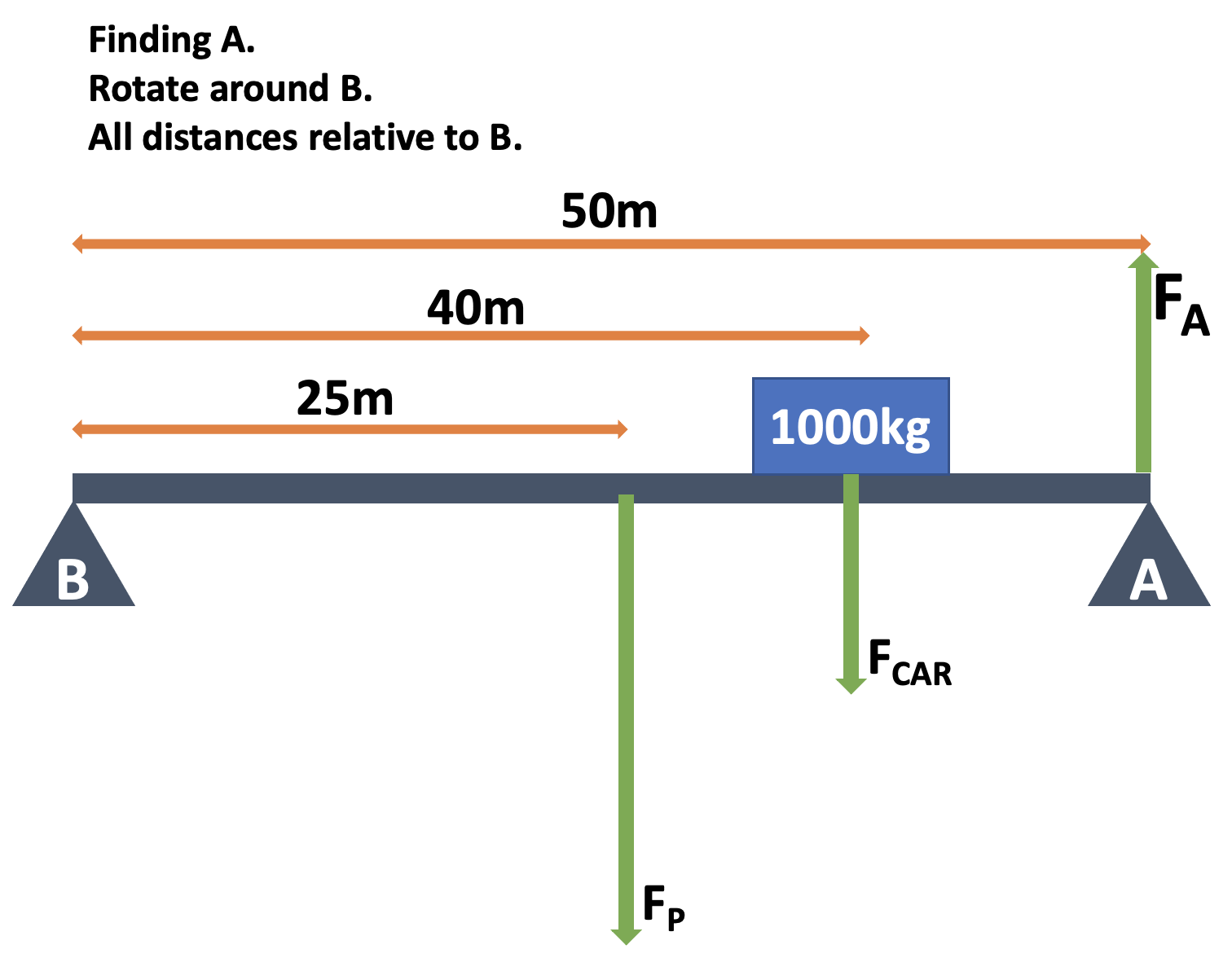
We are assuming the bridge is in equilibrium. This means that both net torque and net force are zero.

\[ \begin{aligned} \tau_{CW} &= \tau_{ACW} \cr \tau_{P} + \tau_{C} &= \tau_{A} \cr F_{P}d_{P} + F_{C}d_{C} &= F_{A}d_{A} \cr (m_{p}g)d_{p} + (m_{c}g)d_{C} &= F_{A}d_{A} \cr ((10,000 \times 9.8) \times 25) + ((1,000 \times 9.8) \times 40) &= F_{A} \times 50 \cr 2,450,000 + 392,000 &= F_{A} \times 50 \cr \frac{2,842,000}{50} &= F_{A} = 56,840N \end{aligned} \]

Whakatika: Support B

We are assuming the bridge is in equilibrium. This means that both net torque and net force are zero.

\[ \begin{aligned} \tau_{CW} &= \tau_{ACW} \cr \tau_{B} &= \tau_{P} + \tau_{C} \cr F_{B}d_{B} &= F_{P}d_{P} + F_{C}d_{C} \cr F_{B}d_{B} &= (m_{P}g)d_{P} + (m_{C}g)d_{C} \cr F_{B} \times 50 &= (10,000 \times 9.8 \times 25) + (1,000 \times 9.8 \times 10) \cr F_{B} \times 50 &= 2450000 + 98000 \cr F_{B} &= \frac{2,548,000}{50} = 50,960N \end{aligned} \]

Ngā Whakaaro / Thoughts

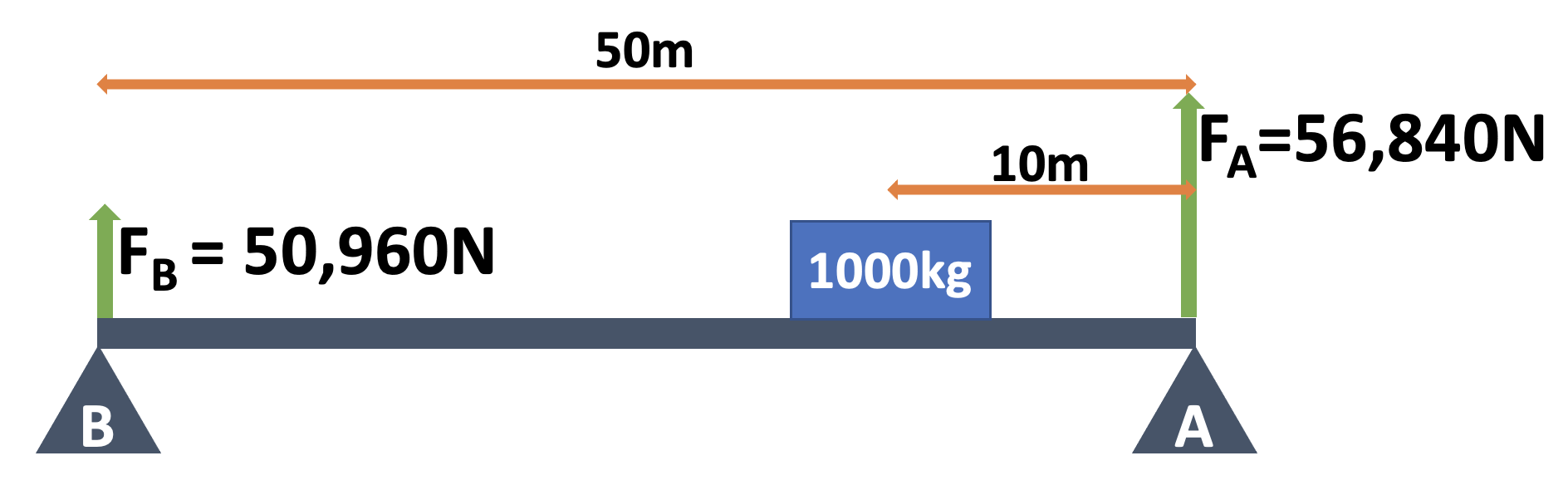
Because the car is closer to support B, it makes logical sense that support B should feel more of the weight force. Therefore, it needs to provide more support force in order to stay in equilibrium.

Whakawai / Practise

Textbook: Force, Equilibrium and Motion - Q7, 8, 10, 11, 12 Homework: Q41, Q43